🔬 Tutorial problems delta \(\delta\)

Note

This problems are designed to help you practice the concepts covered in the lectures. Not all problems may be covered in the tutorial, those left out are for additional practice on your own.

\(\delta\).1

Formulation

Find the (Euclidean) distances between the following pairs of points.

1. \((1, 3)\) and \((2, 4)\)

2. \((-1, 2)\) and \((3, 3)\)

3. \((\tfrac{3}{2},-2)\) and \((-5,1)\)

4. \((x,y)\) and \((2x,y+3)\)

5. \((a, b)\) and \((-a, b)\)

6. \((a,3)\) and \((2+a,5)\)

[Sydsæter, Hammond, Strøm, and Carvajal, 2016] Exercises for Section 5.5, Question 1

Solution

Recall that the distance between two points \((x_1,y_1)\) and \((x_2,y_2)\) is given by \(\sqrt{(x_1-x_2)^2+(y_1-y_2)^2}\).

1. Distance between \((1, 3)\) and \((2, 4)\) is given by \(\sqrt{(1-2)^2+(3-4)^2} = \sqrt{1+1} = \sqrt{2}\)

2. Distance between \((-1, 2)\) and \((3, 3)\) is given by \(\sqrt{(-1-3)^2+(2-3)^2} = \sqrt{(4)^2+(1)^2} = \sqrt{17}\)

3. Distance between \((\tfrac{3}{2},-2)\) and \((-5,1)\) is given by \(\sqrt{(\tfrac{3}{2}-(-5))^2+(-2-1)^2} = \sqrt{(\tfrac{13}{2})^2+(3)^2} = \sqrt{(\tfrac{13}{2})^2+(3)^2} = \sqrt{\tfrac{169}{4} + 9} = \sqrt{\tfrac{205}{4}} = \tfrac{\sqrt{205}}{2}\)

4. Distance between \((x,y)\) and \((2x,y+3)\) is given by \(\sqrt{(x-2x)^2+(y-(y+3))^2} = \sqrt{x^2+3^2} = \sqrt{x^2+9}\)

5. Distance between \((a, b)\) and \((-a, b)\) is given by \(\sqrt{(a-(-a))^2+(b-b)^2} = \sqrt{(2a)^2+0^2} = \sqrt{4a^2} = 2|a|\). We need absolute value because distance is always non-negative.

6. Distance between \((a,3)\) and \((2+a,5)\) is given by \(\sqrt{(a-(2+a))^2+(3-5)^2} = \sqrt{(2)^2+(2)^2} = 2\sqrt{2}\)

\(\delta\).2

Formulation

What are the first five terms of each of the following sequences?

(a) \(\left\{ \frac{2n - 1}{3n+2} \right\}_{n \in \mathbb{N}}\)

(b) \(\left\{ \frac{1 - (-1)^n}{n^3} \right\}_{n \in \mathbb{N}}\)

(c) \(\left\{ \frac{(-1)^{n - 1}}{(2)(4)(6)\cdots(2n)} \right\}_{n \in \mathbb{N}}\)

(d) \(\left\{ \frac{1}{2} + \frac{1}{4} + \frac{1}{8} + \cdots + \frac{1}{2^n}\right\}_{n \in \mathbb{N}}\)

(e) \(\left\{\frac{(-1)^{n - 1} x^{2n-1}}{(2n-1)!} \right\}_{n \in \mathbb{N}}\)

Solution

(a)

The first five terms of the sequence \(\left\{\frac{2 n-1}{3 n+2}\right\}_{n \in \mathbb{N}}\) are

\[\begin{split} \begin{array}{l} x_{1}=\frac{2(1)-1}{3(1)+2}=\frac{2-1}{3+2}=\frac{1}{5}, \\ x_{2}=\frac{2(2)-1}{3(2)+2}=\frac{4-1}{6+2}=\frac{3}{8}, \\ x_{3}=\frac{2(3)-1}{3(3)+2}=\frac{6-1}{9+2}=\frac{5}{11}, \\ x_{4}=\frac{2(4)-1}{3(4)+2}=\frac{8-1}{12+2}=\frac{7}{14}=\frac{1}{2}, \\ x_{5}=\frac{2(5)-1}{3(5)+2}=\frac{10-1}{15+2}=\frac{9}{17} \text {. } \end{array} \end{split}\]

(b) The first five terms of the sequence \(\left\{\frac{1-(-1)^{n}}{n^{3}}\right\}_{n \in \mathbb{N}}\) are

\[\begin{split} \begin{array}{l} x_{1}=\frac{1-(-1)^{1}}{1^{3}}=\frac{1-(-1)}{1}=\frac{1+1}{1}=\frac{2}{1}=2, \\ x_{2}=\frac{1-(-1)^{2}}{2^{3}}=\frac{1-(1)}{8}=\frac{1-1}{8}=\frac{0}{8}=0, \\ x_{3}=\frac{1-(-1)^{3}}{3^{3}}=\frac{1-(-1)}{27}=\frac{1+1}{27}=\frac{2}{27}, \\ x_{4}=\frac{1-(-1)^{4}}{4^{3}}=\frac{1-(1)}{64}=\frac{1-1}{64}=\frac{0}{64}=0, \\ x_{5}=\frac{1-(-1)^{5}}{5^{3}}=\frac{1-(-1)}{125}=\frac{1+1}{125}=\frac{2}{125} . \end{array} \end{split}\]

(c) The first five terms of the sequence \(\left\{\frac{(-1)^{n-1}}{(2)(4)(6) \cdots(2 n)}\right\}_{n \in \mathbb{N}}\) are

\[\begin{split} \begin{array}{l} x_{1}=\frac{(-1)^{1-1}}{(2)}=\frac{(-1)^{0}}{2}=\frac{1}{2}, \\ x_{2}=\frac{(-1)^{2-1}}{(2)(4)}=\frac{(-1)^{1}}{8}=\frac{-1}{8} \\ x_{3}=\frac{(-1)^{3-1}}{(2)(4)(6)}=\frac{(-1)^{2}}{48}=\frac{1}{(48)}, \\ x_{4}=\frac{(-1)^{4-1}}{(2)(4)(6)(8)}=\frac{(-1)^{3}}{384}=\frac{-1}{384}, \\ x_{5}=\frac{(-1)^{5-1}}{(2)(4)(6)(8)(10)}=\frac{(-1)^{4}}{3,840}=\frac{1}{(3,840)} \end{array} \end{split}\]

(d)

The first five terms of the sequence \(\left\{\frac{1}{2}+\frac{1}{4}+\frac{1}{8}+\cdots+\frac{1}{2^{n}}\right\}_{n \in \mathbb{N}}\) are

\[\begin{split} \begin{array}{l} x_{1}=\sum_{i=1}^{1} \frac{1}{2^{i}}=\frac{1}{2^{1}}=\frac{1}{2}, \\ x_{2}=\sum_{i=1}^{2} \frac{1}{2^{i}}=\frac{1}{2^{1}}+\frac{1}{2^{2}}=\frac{1}{2}+\frac{1}{4}=\frac{3}{4} \\ x_{3}=\sum_{i=1}^{3} \frac{1}{2^{i}}=\frac{1}{2^{1}}+\frac{1}{2^{2}}+\frac{1}{2^{3}}=\frac{1}{2}+\frac{1}{4}+\frac{1}{8}=\frac{3}{4}+\frac{1}{8}=\frac{7}{8}, \\ x_{4}=\sum_{i=1}^{4} \frac{1}{2^{i}}=\frac{1}{2^{1}}+\frac{1}{2^{2}}+\frac{1}{2^{3}}+\frac{1}{2^{4}}=\frac{1}{2}+\frac{1}{4}+\frac{1}{8}+\frac{1}{16}=\frac{7}{8}+\frac{1}{16}=\frac{15}{16}, \\ x_{5}=\sum_{i=1}^{5} \frac{1}{2^{i}}=\frac{1}{2^{1}}+\frac{1}{2^{2}}+\frac{1}{2^{3}}+\frac{1}{2^{4}}+\frac{1}{2^{5}}=\frac{1}{2}+\frac{1}{4}+\frac{1}{8}+\frac{1}{16}+\frac{1}{32}=\frac{15}{16}+\frac{1}{32}=\frac{31}{32} . \end{array} \end{split}\]

(e)

The first five terms of the sequence \(\left\{\frac{(-1)^{n-1} x^{2 n-1}}{(2 n-1) !}\right\}_{n \in \mathbb{N}}\) are

\[\begin{split} \begin{array}{l} x_{1}=\frac{(-1)^{1-1} x^{2(1)-1}}{(2(1)-1) !}=\frac{(-1)^{0} x^{2-1}}{((2-1) !}=\frac{(1) x^{1}}{1 !}=\frac{x^{1}}{1}=x, \\ x_{2}=\frac{(-1)^{2-1} x^{2(2)-1}}{(2(2)-1) !}=\frac{(-1)^{1} x^{4-1}}{((4-1) !}=\frac{(-1) x^{3}}{3 !}=\frac{-x^{3}}{(3)(2)(1)}=\frac{-x^{3}}{6}, \\ x_{3}=\frac{(-1)^{3-1} x^{2(3)-1}}{(2(3)-1) !}=\frac{(-1)^{2} x^{6-1}}{((6-1) !}=\frac{(1) x^{5}}{5 !}=\frac{x^{5}}{(5)(4)(3)(2)(1)}=\frac{x^{5}}{120}, \\ x_{4}=\frac{(-1)^{4-1} x^{2(4)-1}}{(2(4)-1) !}=\frac{(-1)^{3} x^{8-1}}{((8-1) !}=\frac{(-1) x^{7}}{7 !}=\frac{-x^{7}}{(7)(6)(5)(4)(3)(2)(1)}=\frac{-x^{7}}{5,040}, \\ x_{5}=\frac{(-1)^{5-1} x^{2(5)-1}}{(2(5)-1) !}=\frac{(-1)^{4} x^{10-1}}{((10-1) !}=\frac{(1) x^{9}}{9 !}=\frac{x^{9}}{(9)(8)(7)(6)(5)(4)(3)(2)(1)}=\frac{x^{9}}{362,880} \end{array} \end{split}\]

\(\delta\).3

Formulation

Consider the following business opportunity. If you pay \(\$ F\) now (in period zero), you can build a production plant that will be ready for operation in the next period. This plant will cost \(\$ C\) per period to operate in any subsequent period (starting from period one). It cannot be operated in period zero, because it has not yet been built. In any period following a period in which the plant was operating, you will have output to sell. The sale of this output yields revenue of \(\$ R\). (You may assume that the retailing process incurs no additional costs. If you prefer, you can think of the revenue in any given period as being net revenue after the subtraction of any retailing costs incurred in that period.) In other words, if the plant is operated in period \(t\), then you will receive \(\$ R\) of revenue in period \((t+1)\). The plant will be worn out after exactly \(n\) periods of operation. Suppose that you decide to build the plant and operate it in all subsequent periods. Note that this means that the plant will begin operation in period one and it will be worn out after (at the end of) period \(n\). Thus the first period in which you will receive revenue is period two and the last period in which you will receive revenue is period \((n+1)\). Suppose that the per-period interest rate is denoted by \(i\) You may assume that this interest rate is fixed over the entire horizon of this project and that \(0<i<1\).

(a) What is the sequence of fixed costs for this project? What is the sequence of operational costs for this project? What is the sequence of revenues from this project? What is the stream of per-period profits for this project?

(b) What is the present value of the fixed cost sequence for this project?

(c) What is the present value of the operational cost sequence for this project?

(d) What is the present value of the revenue sequence for this project?

(e) What is the present value of the profit sequence for this project?

Solution

The timing of the receipts and outlays for this project is illustrated in Table One.

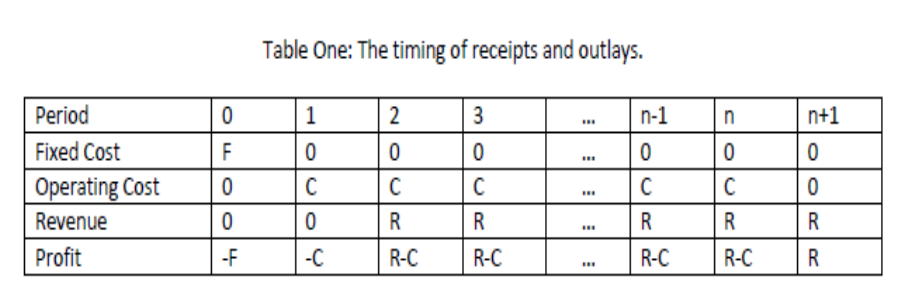
(a) The sequence of fixed costs is

\[ \left\{F_{0}, F_{1}, F_{2}, \cdots, F_{n+1}\right\}=\{F, 0,0, \cdots, 0\} \]

The sequence of operating costs is

\[ \left\{C_{0}, C_{1}, C_{2}, \cdots, C_{n}, C_{n+1}\right\}=\{0, C, C, \cdots, C, 0\} \]

The sequence of revenues for this project is

\[ \left\{R_{0}, R_{1}, R_{2}, R_{3}, \cdots, R_{n+1}\right\}=\{0,0, R, R, \cdots, R\} \]

The sequence of profits for this project is

\[ \left\{\pi_{0}, \pi_{1}, \pi_{2}, \pi_{3}, \cdots, \pi_{n}, \pi_{n+1}\right\}=\{-F,-C, R-C, R-C, \cdots, R-C, R\} \]

(b)

The present value of the fixed cost sequence for this project is

\[\begin{split} \begin{array}{l} P V(F) =\sum_{t=0}^{n+1} \frac{F_{t}}{(1+i)^{t}} \\ =\frac{F_{0}}{(1+i)^{0}}+\sum_{t=1}^{n+1} \frac{F_{t}}{(1+i)^{t}} \\ =\frac{F}{1}+\sum_{t=1}^{n+1} \frac{0}{(1+i)^{t}} \\ =F+0 \\ =F . \end{array} \end{split}\]

(c)

The present value of the operational cost sequence for this project is

\[\begin{split} \begin{array}{l} P V(C) =\sum_{t=0}^{n+1} \frac{C_{t}}{(1+i)^{t}} \\ =\frac{C_{0}}{(1+i)^{0}}+\sum_{t=1}^{n} \frac{C_{t}}{(1+i)^{t}}+\frac{C_{n+1}}{(1+i)^{n}} \\ =\frac{0}{1}+\frac{1}{(1+i)} \sum_{t=1}^{n} \frac{C}{(1+i)^{t-1}}+\frac{0}{(1+i)^{n}} \\ =0+\frac{1}{(1+i)} \sum_{k=0}^{n-1} \frac{C}{(1+i)^{k}}+0 \\ =\frac{C}{(1+i)} \sum_{k=0}^{n-1}\left(\frac{1}{1+i}\right)^{k} \\ =\frac{C}{(1+i)}\left\{\frac{1\left(1-\left(\frac{1}{1+i}\right)^{n}\right)}{1-\left(\frac{1}{1+i}\right)}\right\} \\ =\frac{C}{(1+i)}\left\{\frac{\left(1-\left(\frac{1}{1+i}\right)^{n}\right)}{\left(\frac{1+i-1}{1+i}\right)}\right\} \\ =\frac{C}{(1+i)}\left\{\frac{\left(1-\left(\frac{1}{1+i}\right)^{n}\right)}{\left(\frac{1+i - 1}{1+i}\right)}\right\} \\ =\frac{C}{(1+i)}\left\{\frac{\left(1-\left(\frac{1}{1+i}\right)^{n}\right)}{\left(\frac{i}{1+i}\right)}\right\} \\ =\frac{C(1+i)}{i(1+i)}\left(1-\left(\frac{1}{1+i}\right)^{n}\right) \\ =\left(\frac{C}{i}\right)\left(1-\left(\frac{1}{1+i}\right)^{n}\right) . \end{array} \end{split}\]

(d)

The present value of the revenue sequence for this project is

\[\begin{split} \begin{array}{l} P V(R)=\sum_{t=0}^{n+1} \frac{R_{t}}{(1+i)^{t}} \\ =\frac{R_{0}}{(1+i)^{0}}+\frac{R_{1}}{(1+i)^{1}}+\sum_{t=2}^{n+1} \frac{R_{t}}{(1+i)^{t}} \\ =\frac{0}{1}+\frac{0}{(1+i)}+\sum_{t=2}^{n+1} \frac{R}{(1+i)^{t}} \\ =0+0+\frac{1}{(1+i)^{2}} \sum_{t=2}^{n+1} \frac{R}{(1+i)^{t-2}} \\ =\frac{1}{(1+i)^{2}} \sum_{k=0}^{n-1} \frac{R}{(1+i)^{k}} \\ =\frac{R}{(1+i)^{2}} \sum_{k=0}^{n-1} \frac{1}{(1+i)^{k}} \\ =\frac{R}{(1+i)^{2}} \sum_{k=0}^{n-1}\left(\frac{1}{1+i}\right)^{k} \\ =\frac{R}{(1+i)^{2}}\left\{\frac{1\left(1-\left(\frac{1}{1+i}\right)^{n}\right)}{1-\left(\frac{1}{1+i}\right)}\right\} \\ =\frac{R}{(1+i)^{2}}\left\{\frac{\left(1-\left(\frac{1}{1+i}\right)^{n}\right)}{\left(\frac{1+i-1}{1+i}\right)}\right\} \\ =\frac{R}{(1+i)^{2}}\left\{\frac{\left(1-\left(\frac{1}{1+i}\right)^{n}\right)}{\left(\frac{1+i-1}{1+i}\right)}\right\} \\ =\frac{R}{(1+i)^{2}}\left\{\frac{\left(1-\left(\frac{1}{1+i}\right)^{n}\right)}{\left(\frac{i}{1+i}\right)}\right\} \\ =\frac{R(1+i)}{i(1+i)^{2}}\left(1-\left(\frac{1}{1+i}\right)^{n}\right) \\ =\left(\frac{R}{i(1+i)}\right)\left(1-\left(\frac{1}{1+i}\right)^{n}\right) . \end{array} \end{split}\]

(e)

The present value of the revenue sequence for this project is

\[\begin{split} \begin{array}{l} P V(\pi)=\sum_{t=0}^{n+1} \frac{\pi_{t}}{(1+i)^{t}} \\ =\frac{\pi_{0}}{(1+i)^{0}}+\frac{\pi_{1}}{(1+i)^{1}}+\sum_{t=2}^{n} \frac{\pi_{t}}{(1+i)^{t}}+\frac{\pi_{n}}{(1+i)^{n+1}} \\ =\frac{-F}{1}+\frac{-C}{(1+i)}+\sum_{t=2}^{n} \frac{(R-C)}{(1+i)^{t}}+\frac{R}{(1+i)^{n+1}} \\ =\left\{\frac{R}{(1+i)^{n+1}}-\frac{C}{(1+i)}-F\right\}+\frac{(R-C)}{(1+i)^{2}} \sum_{t=2}^{n} \frac{1}{(1+i)^{t-2}} \\ =\left\{\frac{R}{(1+i)^{n+1}}-\frac{C}{(1+i)}-F\right\}+\frac{(R-C)}{(1+i)^{2}} \sum_{t=2}^{n}\left(\frac{1}{1+i}\right)^{t-2} \\ =\left\{\frac{R}{(1+i)^{n+1}}-\frac{C}{(1+i)}-F\right\}+\frac{(R-C)}{(1+i)^{2}} \sum_{k=0}^{n-2}\left(\frac{1}{1+i}\right)^{k} \\ =\left\{\frac{R}{(1+i)^{n+1}}-\frac{C}{(1+i)}-F\right\}+\frac{(R-C)}{(1+i)^{2}}\left\{\frac{1\left(1-\left(\frac{1}{1+i}\right)^{n-1}\right)}{1-\left(\frac{1}{1+i}\right)}\right\} \\ =\left\{\frac{R}{(1+i)^{n+1}}-\frac{C}{(1+i)}-F\right\}+\frac{(R-C)}{(1+i)^{2}}\left\{\frac{\left(1-\left(\frac{1}{1+i}\right)^{n-1}\right)}{\left(\frac{1+i-1}{1+i}\right)}\right\} \\ =\left\{\frac{R}{(1+i)^{n+1}}-\frac{C}{(1+i)}-F\right\}+\frac{(R-C)}{(1+i)^{2}}\left\{\frac{\left(1-\left(\frac{1}{1+i}\right)^{n-1}\right)}{\left(\frac{i}{1+i}\right)}\right\} \\ =\left\{\frac{R}{(1+i)^{n+1}}-\frac{C}{(1+i)}-F\right\}+\frac{(R-C)(1+i)}{i(1+i)^{2}}\left\{1-\left(\frac{1}{1+i}\right)^{n-1}\right\} \\ =\left\{\frac{R}{(1+i)^{n+1}}-\frac{C}{(1+i)}-F\right\}+\frac{(R-C)}{i(1+i)}\left\{1-\left(\frac{1}{1+i}\right)^{n-1}\right\} . \end{array} \end{split}\]

Note that this can be rewritten as

\[\begin{split} \begin{array}{l} P V(\pi)=\frac{R}{(1+i)^{n+1}}-\frac{C}{(1+i)}-F+\frac{R}{i(1+i)}\left\{1-\left(\frac{1}{1+i}\right)^{n-1}\right\} -\frac{C}{i(1+i)}\left\{1-\left(\frac{1}{1+i}\right)^{n-1}\right\} \\ =\frac{R}{i(1+i)}\left\{\frac{i}{(1+i)^{n}}+1-\left(\frac{1}{1+i}\right)^{n-1}\right\} -\frac{C}{i(1+i)}\left\{i+1-\left(\frac{1}{1+i}\right)^{n-1}\right\}-F \\ =\frac{R}{i(1+i)}\left\{\frac{i}{(1+i)^{n}}+1-\frac{1}{(1+i)^{n-1}}\right\} -\frac{C}{i(1+i)}\left\{(1+i)-\frac{1}{(1+i)^{n-1}}\right\}-F \\ =\frac{R}{i(1+i)}\left\{\frac{i}{(1+i)^{n}}+\frac{(1+i)^{n}}{(1+i)^{n}}-\frac{(1+i)}{(1+i)^{n}}\right\} -\frac{C}{i}\left\{\frac{(1+i)}{(1+i)}-\frac{1}{(1+i)^{n}}\right\}-F \\ =\frac{R}{i(1+i)}\left\{\frac{i+(1+i)^{n}-(1+i)}{(1+i)^{n}}\right\} -\frac{C}{i}\left\{1-\frac{1}{(1+i)^{n}}\right\}-F \\ =\frac{R}{i(1+i)}\left\{\frac{i+(1+i)^{n}-1-i}{(1+i)^{n}}\right\} -\frac{C}{i}\left\{1-\frac{1}{(1+i)^{n}}\right\}-F \\ =\frac{R}{i(1+i)}\left\{\frac{(1+i)^{n}-1}{(1+i)^{n}}\right\}-\frac{C}{i}\left\{1-\frac{1}{(1+i)^{n}}\right\}-F \\ =\frac{R}{i(1+i)}\left\{\frac{(1+i)^{n}}{(1+i)^{n}}-\frac{1}{(1+i)^{n}}\right\}-\frac{C}{i}\left\{1-\frac{1}{(1+i)^{n}}\right\}-F \\ =\frac{R}{i(1+i)}\left\{1-\left(\frac{1}{1+i}\right)^{n}\right\}-\frac{C}{i}\left\{1-\left(\frac{1}{1+i}\right)^{n}\right\}-F \\ =P V(R)-P V(C)-P V(F) . \end{array} \end{split}\]

\(\delta\).4

Formulation

Compute the following limits:

1. \(\quad \lim_{n \to \infty} \frac{1}{n}\)

2. \(\quad \lim_{n \to \infty} \frac{n+2}{2n+1}\)

3. \(\quad \lim_{n \to \infty} \frac{2n^2(n-2)}{(1-3n)(2+n^2)}\)

4. \(\quad \lim_{n \to \infty} \frac{(n+1)!}{n! - (n+1)!}\)

5. \(\quad \lim_{n \to \infty} \sqrt{\frac{9+n^2}{4n^2}}\)

Hint

Use the properties of the limit where possible and only resort to the definition when necessary.

Solution

1.

Let’s prove that \(\lim_{n \to \infty} \frac{1}{n} = 0\) using the definition of a limit.

First pick an arbitrary \(\epsilon > 0\). Now we have to come up with an \(N\) such that $\( n \geq N \implies |1/n - 0| < \epsilon \)$

Let \(N\) be the first integer greater than \(1/\epsilon\). Then $\( n \geq N \implies n > 1/\epsilon \implies 1/n < \epsilon \implies |1/n - 0| < \epsilon \)$

2.

\[\begin{split} \begin{array}{l} \lim_{n \to \infty} \frac{n+2}{2n+1} = \lim_{n \to \infty} \frac{1+2/n}{2+1/n} = \\ = \frac{1+\lim_{n \to \infty}2/n}{2+\lim_{n \to \infty}1/n} = \frac{1+0}{2+0} = \frac{1}{2} \end{array} \end{split}\]

3.

\[\begin{split} \begin{array}{l} \lim_{n \to \infty} \frac{2n^2(n-2)}{(1-3n)(2+n^2)} = \lim_{n \to \infty} \frac{2n^3-4n^2}{2+n^2-6n-3n^3} = \\ = \lim_{n \to \infty} \frac{2-4/n}{2/n^3+1/n-6/n^2-3} = \frac{2-\lim_{n \to \infty}4/n}{\lim_{n \to \infty}2/n^3+\lim_{n \to \infty}1/n-\lim_{n \to \infty}6/n^2-3} = \\ = \frac{2-0}{0+0-0-3} = -\frac{2}{3} \end{array} \end{split}\]

4.

Note that the factorial operation is defined as \((n+1)! = (n+1)n(n-1)\cdot .. \cdot 1\)

\[\begin{split} \begin{array}{l} \lim_{n \to \infty} \frac{(n+1)!}{n! - (n+1)!} = \lim_{n \to \infty} \frac{(n+1)n!}{n!(1- (n+1))} = \\ = \lim_{n \to \infty} \frac{n+1}{1- n -1} = \lim_{n \to \infty} \frac{1+1/n}{(-1)} = \\ = \frac{1+\lim_{n \to \infty} 1/n}{(-1)} = -\frac{1+0}{1} = -1 \end{array} \end{split}\]

5.

\[\begin{split} \begin{array}{l} \lim_{n \to \infty} \sqrt{\frac{9+n^2}{4n^2}} = \sqrt{\lim_{n \to \infty} \frac{9/n^2+1}{4}} = \\ = \sqrt{\frac{\lim_{n \to \infty}9/n^2+1}{4}} = \sqrt{\frac{0+1}{4}} = \frac{1}{2} \end{array} \end{split}\]

\(\delta\).5

Formulation

Use the squeeze theorem to compute the following limits:

1. \(\lim_{n \to \infty} f(n) \; \text{where} \; 1 \leqslant f(n) \leqslant \frac{n}{n+2}\)

2. \(\lim_{n \to \infty} \frac{\cos(n)}{n^3}\)

3. \(\lim_{n \to \infty} \Big[ \frac{1}{n^2} \sin(3n) + 2 \Big]\)

Solution

1.

\(\lim_{n \to \infty} f(n) \; \text{where} \; 1 \leqslant f(n) \leqslant \frac{n}{n+2}\)

Note that

\[ \lim_{n \to \infty} \frac{n}{n+2} = \lim_{n \to \infty} \frac{1}{1+\tfrac{2}{n}} = \frac{1}{1+\lim_{n \to \infty}\tfrac{2}{n}} = \frac{1}{1+0} = 1 \]

Assuming that \(f(n)\) is convergent, by the preservation of inequalities under limits, we conclude that \(\lim_{n \to \infty} f(n) \geqslant 1\) and \(\lim_{n \to \infty} f(n) \leqslant 1\), thus \(\lim_{n \to \infty} f(n) = 1\). The existence of the limit of \(f(n)\) is guaranteed by the squeeze theorem.

2.

\(\lim_{n \to \infty} \frac{\cos(n)}{n^3}\)

\[\begin{split} \begin{array}{rcl} -1 \leqslant& \cos(n) &\leqslant 1 \\ -\frac{1}{n^3} \leqslant& \frac{\cos(n)}{n^3} &\leqslant \frac{1}{n^3} \\ \end{array} \end{split}\]

Taking the limits of the left and right sides of the inequality as \(n \to \infty\), we have

\[ -\frac{1}{n^3} \to 0, \quad \frac{1}{n^3} \to 0 \]

By the squeeze theorem, we then have $\( \lim_{n \to \infty} \frac{\cos(n)}{n^3} = 0 \)$

3.

\(\lim_{n \to \infty} \Big[ \frac{1}{n^2} \sin(3n) + 2 \Big]\)

\[\begin{split} \begin{array}{rcl} -1 \leqslant& \sin(3n) &\leqslant 1 \\ -\frac{1}{n^2} \leqslant& \frac{\sin(3n)}{n^2} &\leqslant \frac{1}{n^2} \\ -\frac{1}{n^2} +2 \leqslant& \frac{\sin(3n)}{n^2}+2 &\leqslant \frac{1}{n^2} +2 \end{array} \end{split}\]

Taking the limits of the left and right sides of the inequality as \(n \to \infty\), we have

\[ -\frac{1}{n^2} +2 \to 2, \quad \frac{1}{n^2} +2 \to 2 \]

By the squeeze theorem, we then have \(\lim_{n \to \infty} \Big[ \frac{1}{n^2} \sin(3n) + 2 \Big] = 2\)